搜索结果: 1-15 共查到“几何学”相关记录2076条 . 查询时间(3.598 秒)
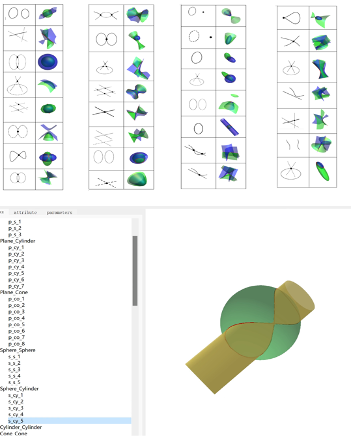
CAD几何内核核心算法库（图）
CAD 几何内核 核心算法
2024/10/29
该成果是CAD几何内核的核心底层技术，很大程度上决定了几何引擎的稳定性。二次曲面在CAD模型中使用最为广泛，因此二次曲面的求交在所有求交任务中被调用频次最高。由于二次曲面具有特殊的代数和几何性质，它们的求交计算一般需区别于自由曲面求交来进行特殊处理，从而充分保证算法的精度和效率。
教育部数学“101计划”几何类课程建设研讨会在合肥召开（图）
101计划 几何 课程建设研讨会
2024/9/3
为贯彻落实教育部关于教育教学改革系列“101计划”，进一步推进数学学科人才培养改革，加强几何方向核心课程建设，2024年6月1日，“101计划”几何课程研讨会在中国科学技术大学举办。
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Nonlinear potentials at the fractional scale:sharp regularity
分数尺度 非线性势 尖锐 正则性
2023/11/29
Nonlinear potential theory and elliptic regularity theory are two classical topics in the modern analysis of partial differential equations. In this talk I show how these themes merge to solve the lon...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Metric geometric aspects of Einstein manifolds
Einstein流形 度量几何 爱因斯坦流形 黎曼几何
2023/11/29
This lecture concerns the metric Riemannian geometry of Einstein manifolds, which is a central theme in modern differential geometry and is deeply connected to a large variety of fundamental problems ...
荆楚理工学院数理学院硕士生导师邓严林教授
荆楚理工学院数理学院 硕士生导师 教授 微分几何 几何分析
2024/4/19
邓严林，教授、硕士生导师，现任荆楚理工学院教师工作部部长、人事处处长。
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Kahler geometry methods in Gaussian Gabor frame theory
高斯 Gabor框架理论 Kahler几何
2023/11/6
We investigate the frame set of regular multivariate Gaussian Gabor frames using methods from Kahler geometry such as Hormander's $\dbar$-L2 estimate with singular weight, Demailly's Calabi--Yau metho...
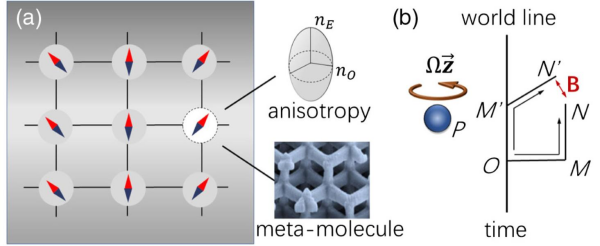
中国科学院半导体研究所在非互易光学介质几何理论方面取得进展（图）
非互易 光学介质 几何理论
2023/5/24
光在复杂介质中的传播是光学和相对论的经典课题。在爱因斯坦提出广义相对论不久，W. Gordon，I. E. Tamm和G. V. Skrotskii等将费马原理推广到弯曲时空。1960年，J. Plebanski指出弯曲时空度规的空间分量和时空混合分量分别等价于非均匀各向异性光学介质的折射率(介电常数与磁导率)和反对称非互易磁电耦合参数。上述结果已被广泛应用于引力场量子效应的实验室模拟。2006年...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Almost sure scattering for the cubic NLS on R3T1
R3T1 三次NLS 散射 Cauchy问题
2023/5/18
We consider the Cauchy problem for the defocusing cubic NLS on R3T1 and establish almost sure scattering for random initial data. The main obstacle to extend the classical almost sure scattering resul...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Hausdorff dimension, singular vectors and divergent trajectories
豪斯多夫维数 奇异向量 发散轨迹
2023/5/12
In this talk, I will give a survey of results related to the problem of computing Hausdorff dimension of various dynamically defined sets, such as singular vectors. The aim is to try to describe the l...
CAD几何引擎是核心工业软件的卡脖子技术，而参数曲面求交又是CAD几何引擎中最核心的问题。参数曲面求交面临的挑战主要是算法的稳定性，交线的精度控制和求交的效率。
本工作主要是对参数曲面求交稳定性问题展开研究，首次给出了两个参数曲面交线的完全的拓扑结构分析和可行的计算方法，为开发稳定的CAD几何引擎奠定了理论基础。文章被计算机图形学顶会接收。
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Kazdan-Warner equations on compact Riemann surfaces
紧黎曼曲面 卡兹丹-华纳方程 数学
2023/4/28
In this talk, we shall review firstly the study history of Kazdan-Warner equations on compact Riemann surfaces, which was proposed by Kazdan and Warner on Annals of Math on 1974. Then we shall show th...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：The logarithmic Brunn-Minkowski inequality conjecture in convex geometry
凸几何 对数 布伦-闵可夫斯基 不等式猜想
2023/4/21
The logarithmic Brunn-Minkowski inequality conjecture is one of the most intriguing challenges in convex geometry since 2012. Notably, this conjectured inequality is stronger than the celebrated Brunn...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：On the Riemann-Hilbert problem of confluent hypergeometric systems and Seiberg-Witten curves
超几何系统 塞伯格-维滕曲线 黎曼-希尔伯特问题
2023/4/21
This talk first solves explicitly a Riemann-Hilbert problem of confluent hypergeometric systems. It then conjectures and proves a special case that the WKB approximation of the monodromy data of confl...